I have decided!

I have decided to restart my Calculus learning. Reference book is ‘Introduction to Calculus and Analysis’ by Richard Courant and Fritz John.

Introduction

How to represent rational numbers

For better understand numbers, we need to represent the rational numbers at first.
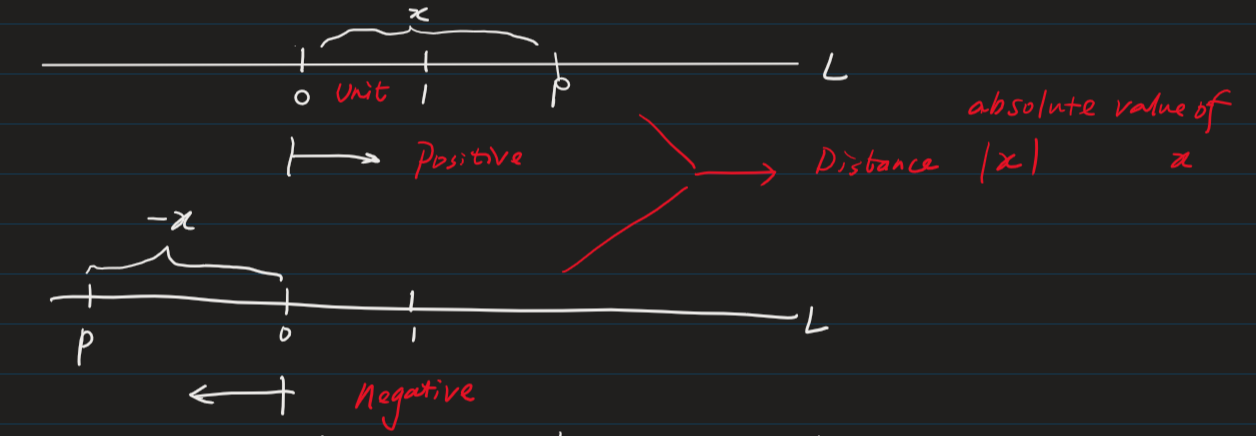
Imagine a number axis L, with a unit length 1, we can rule the positive direction and negative direction.
And then we need to represent the rational numbers. Arbitrarily set a point ‘P’. Segment arbitrary unit, like +- 1, +-2 into smaller pieces, and one of the pieces is 1/p. As you can see in the Figure 2 below.
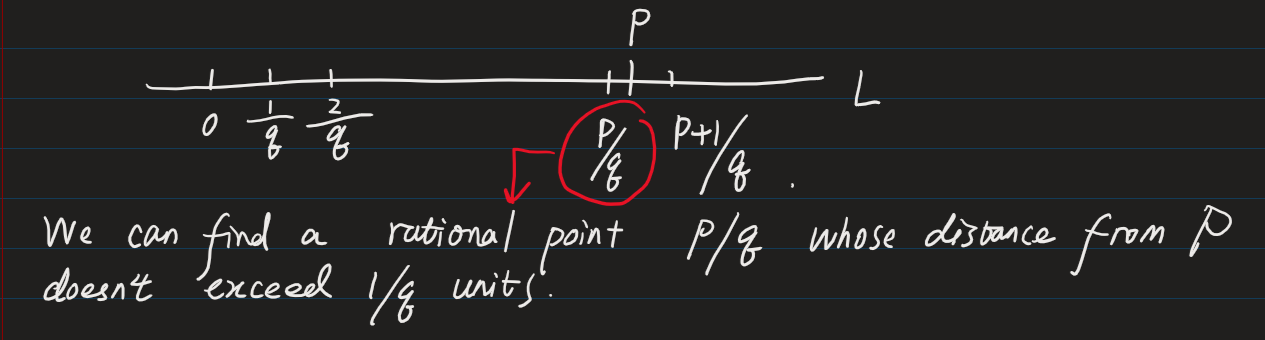In this way, we can find the every point rational point p/q, the P is on the p/q or between two rational point p/q and (p+1)/q. In the other word, we find any rational point p/q on this number axis L.
We call this two quantities whose ratio is a rational number Commensurable Number. Because it can be expressed as integral multiples of a common unit. And there is Incommensurable Number too. But why?

What is irrational number?

Imagine there is triangle with (1^2 + 1^2 = 2)